Electric field intensity

What is the electric field intensity?

The area around a charged body where any other charged body experiences attraction or repulsion is called the electric field of that charge. The force experienced by a unit positive charge placed at any point in an electric field is called the electric field intensity. Electric Field Intensity is a vector quantity. We denote it by ‘E’

If we place +q charge at any point of an electric field and it experience F force then the electric intensity of that point E=F/q. The unit of ‘E’ is `NC^{-1}`

Electric intensity is the force per unit positive charge hence electric intensity has direction and it is a vector quantity. The direction of electric intensity is in the direction in which the unit positive charge experience the force.

`\vec E=\frac{\vec F}q`

A is a positively charged body; hence the positive test charge placed at p will experience a repulsive force along with PB. Thus, the direction of electric intensity at p will be along with PB. But if body A is negatively charged, then the test charge placed at p will experience an attraction force along with PA. Thus, the direction of electric intensity at p will be along with PA.

From the equation E= F/q it is seen that dividing the unit of force by unit of charge, unit of electric intensity is found. This unit is newton per coulomb or `NC^{-1}`. The electric intensity at a point is 100N/C means that if we place a 1C charge at the point it will experience 100N force.

Relation Between Force and Intensity:

From the equation, we see that magnitude of the force on a charge placed at a point in the electric field is,

`\vec F=q\vec E`

Or, F=qE

That is the force on a charge placed at a point in the electric field is equal to the product of the electric intensity of the point and the charge. Positive charge experience force along the direction of the intensity and negative charge opposite to the direction of the intensity.

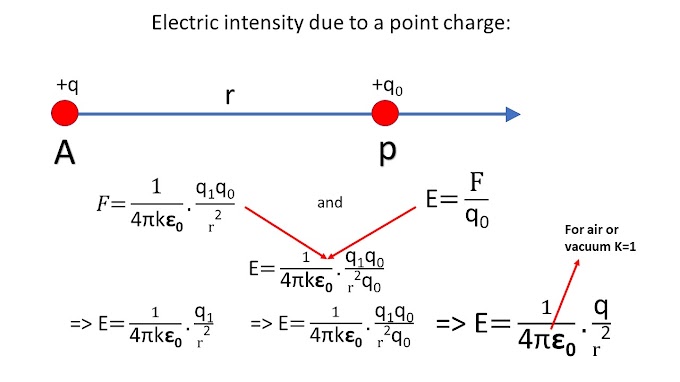
Electric Field intensity due to a point charge:

Let a positive charge +q situated at a point A in a medium whose dielectric constant is k. Now we will determine the electric intensity at a point p at a distance r from this charge.

Suppose a small charge `+q_0` is placed at point P. Now, the force acting on charge `q_0` is

`F=\frac1{4\pi\varepsilon_0k}\frac{qq_0}{r^2}`

But the electric intensity is the force acting on unit charge. Hence electric intensity at point p is

`E=\frac F{q_0}`

Substituting F from the equation we get,

`E=\frac1{4\pi\varepsilon_0k}\frac{qq_0}{r^2q_0}`

Or, `E=\frac{1q}{4\pi\varepsilon_0kr^2}`

If the charge +q is in the vacuum or in the air then k is taken as 1. In that case, the electric intensity would be

`E=\frac1{4\pi\varepsilon_0}\frac q{r^2}`

E is a vector quantity. Its direction would be along the straight-line joining A and P. If q is positive then outwards that is along with PB and if q is negative then inwards that is along with PA.

Principle of superposition of Electric Intensity:

If there are a number of charges in a region of space, then the resultant intensity at any point due to the electric field produced by all the charges can be obtained by taking the vector sum of the individual intensity produced by each charge. This is known as the principle of superposition of electric intensity. If at any point the intensities produced by the charge `q_{1,}q_{2,}q_3`… etc. are respectively. `\vec{E_{1,}}\vec{E_{2,}}\vec{E_3}`…… etc. then the resultant intensity `\vec E` of that point will be

`\vec E=\vec{E_1}+\vec{E_2}+\vec{E_3}+.............=\sum\vec{E_n}`